How To Find The Initial Volume Of A Gas

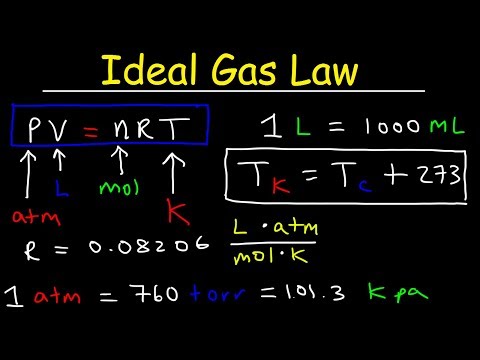
The behavior of gases can be described by several laws based on experimental observations of their properties. The pressure of a given amount of gas is directly proportional to its absolute temperature, provided that the volume does not change (Amontons's law). The volume of a given gas sample is directly proportional to its absolute temperature at constant pressure (Charles's law). The volume of a given amount of gas is inversely proportional to its pressure when temperature is held constant (Boyle's law). Under the same conditions of temperature and pressure, equal volumes of all gases contain the same number of molecules (Avogadro's law). Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures.

We will consider the key developments in individual relationships , then put them together in the ideal gas law. This relationship between temperature and pressure is observed for any sample of gas confined to a constant volume. An example of experimental pressure-temperature data is shown for a sample of air under these conditions in Figure 9.11. Gases whose properties of P, V, and T are accurately described by the ideal gas law are said to exhibit ideal behavior or to approximate the traits of an ideal gas.

An ideal gas is a hypothetical construct that may be used along with kinetic molecular theory to effectively explain the gas laws as will be described in a later module of this chapter. Although all the calculations presented in this module assume ideal behavior, this assumption is only reasonable for gases under conditions of relatively low pressure and high temperature. In the final module of this chapter, a modified gas law will be introduced that accounts for the non-ideal behavior observed for many gases at relatively high pressures and low temperatures. And is a proportionality constant that relates the values of pressure, volume, amount, and temperature of a gas sample.

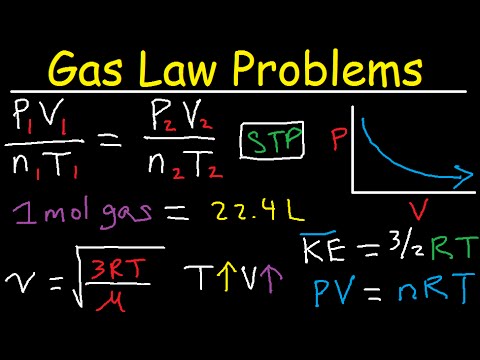
The variables in this equation do not have the subscripts i and f to indicate an initial condition and a final condition. The ideal gas law relates the four independent properties of a gas under any conditions. If a sample of gas has an initial pressure of 375 torr and an initial volume of 7.02 L, what is the final pressure if the volume is changed to 4,577 mL?

Assume that amount and the temperature of the gas remain constant. The volume and temperature are linearly related for 1 mole of methane gas at a constant pressure of 1 atm. If the temperature is in kelvin, volume and temperature are directly proportional.

Charles's law states that the volume of a given amount of gas is directly proportional to its temperature on the kelvin scale when the pressure is held constant. If a sample of gas has an initial pressure of 1.56 atm and an initial volume of 7.02 L, what is the final volume if the pressure is changed to 1,775 torr? Assume that the amount and the temperature of the gas remain constant. Of the three states of matter, gases undergo the greatest volume changes with changing temperature and pressure conditions, but liquids also undergo changes. Liquids aren't responsive to pressure changes, but they can be responsive to temperature changes, depending on their composition. To calculate the volume change of a liquid with respect to temperature, you need to know its coefficient of volumetric expansion.

Gases, on the other hand, all expand and contract more or less in accordance with the ideal gas law, and the volume change is not dependent on its composition. If a sample of gas has an initial pressure of 3.66 atm and an initial volume of 11.8 L, what is the final pressure if the volume is reduced to 5.09 L? Calculate volume change of a liquid with changing temperature by looking up its coefficient of expansion (β) and using the equation. Both the temperature and pressure of a gas are dependent on temperature, so to calculate volume change, use the ideal gas law.

If a sample of gas has an initial pressure of 1.56 atm and an initial volume of 7.02 L, what is the final volume if the pressure is reduced to 0.987 atm? If we partially fill an airtight syringe with air, the syringe contains a specific amount of air at constant temperature, say 25 °C. This example of the effect of volume on the pressure of a given amount of a confined gas is true in general. Decreasing the volume of a contained gas will increase its pressure, and increasing its volume will decrease its pressure. In fact, if the volume increases by a certain factor, the pressure decreases by the same factor, and vice versa.

Volume-pressure data for an air sample at room temperature are graphed in Figure 9.13. Volume-pressure data for an air sample at room temperature are graphed in Figure 5. To apply this gas law, the amount of gas should remain constant.

As with the other gas laws, the temperature must be expressed in kelvins, and the units on the similar quantities should be the same. Because of the dependence on three quantities at the same time, it is difficult to tell in advance what will happen to one property of a gas sample as two other properties change. Although Boyle's law describes the behavior of an ideal gas, it can be applied to real gases at a normal temperature and low pressure.

As temperature and pressure increase, gases start to deviate from any variation of the ideal gas law. This law applies only to ideal gases held at a constant temperature, allowing only the volume and pressure to change. If a gas has an initial pressure of 24,650 Pa and an initial volume of 376 mL, what is the final volume if the pressure of the gas is changed to 775 torr? Imagine filling a rigid container attached to a pressure gauge with gas and then sealing the container so that no gas may escape.

If the container is cooled, the gas inside likewise gets colder and its pressure is observed to decrease. Since the container is rigid and tightly sealed, both the volume and number of moles of gas remain constant. If we heat the sphere, the gas inside gets hotter (Figure 9.10) and the pressure increases. The ideal gas equation contains five terms, the gas constant R and the variable properties P, V, n, and T. Specifying any four of these terms will permit use of the ideal gas law to calculate the fifth term as demonstrated in the following example exercises.

If we heat the sphere, the gas inside gets hotter and the pressure increases. Boyle's law, together with Charles's law and Gay-Lussac's law, are among the fundamental laws which describe the vast majority of thermodynamic processes. We have gathered them all in our thermodynamic processes calculator, where you can choose whichever process you like, and evaluate the outcomes for a real gases. What is its volume if the temperature is changed to −35°C? Assume that the pressure and the amount of the gas remain constant.

What is its volume if the temperature is changed to 60°C? What was the initial volume of the gas in the following case? I have an unknown volume of gas held at a temperature of 115 K in a container with a pressure of 60 atm. What Boyle's law means is that the volume of a mass of gas is inversely proportional to its pressure. This linear relationship between pressure and volume means doubling the volume of a given mass of gas decreases its pressure by half. We can apply the ideal gas law to relate the pressure and volume of state 1 to temperature at state 1.

There are 4 general laws that relate the 4 basic characteristic properties of gases to each other. While it is important to understand the relationships covered by each law, knowing the originator is not as important and will be rendered redundant once the combined gas law is introduced. So concentrate on understanding the relationships rather than memorizing the names. The fuel and air gases in the cylinders of a 1200 cm3 car engine go from 25oC before combustion and rise to a peak temperature of 2100oC after combustion.

If normal atmospheric pressure is 101kPa, calculate the peak pressure reached after combustion. Although the movement of the piston changes the volume, for the sake of argument assume the volume is constant. This resulted in the formulation of the laws of gases described in the next section 4a. Along with how to use them in calculations and problem solving.

Use the ideal gas law calculator to find the pressure, volume and temperature of a gas. Charles' law, together with Boyle's law and Gay-Lussac's law, are among the fundamental laws which describe the vast majority of thermodynamic processes. Experience has shown that several properties of a gas can be related to each other under certain conditions. The properties are pressure , volume , temperature , and amount of material expressed in moles .

What we find is that a sample of gas cannot have any random values for these properties. Instead, only certain values, dictated by some simple mathematical relationships, will occur. Under these conditions, the volume of the gas will vary inversely with the absolute pressure. This equation calculates a pressure given the corresponding elements of the equivalence; Initial pressure, Initial volume, and temperature. For a constant volume and amount of air, the pressure and temperature are directly proportional, provided the temperature is in kelvin.

12.0 dm3 of gas in a cylinder and piston system is heated from 290 K to 340 K. If the pressure remains constant, calculate the final volume of gas in the cylinder. The particle theory of gas pressure was explained in Part 1 so this section concentrates on the gas law calculations involving pressure and volume and their variation with temperature. Boyle's law describes all processes for which temperature remains constant. In thermodynamics, temperature is a measure of the average kinetic energy that atoms or molecules have. In other words, we can say that the average velocity of gas particles doesn't change during that transition.

Boyle's law formula is valid for a wide range of temperatures. Boyle's law describes the behavior of an ideal gas during an isothermal process, which means that the temperature of gas remains constant during the transition, as does the internal energy of the gas. Boyle's law (also known as Boyle-Mariotte law) tells us about the relationship between the pressure of a gas and its volume at a constant temperature and mass of gas. It states that the absolute pressure is inversely proportional to the volume. Balloon flight - you must have seen a balloon in the sky at least once in your life. Have you ever wondered how is it possible for it to fly, and why they are equipped with fire or another heating sources on board?

As a result, the same amount of gas occupies a greater space, which means that the density decreases. The buoyancy of the surrounding air does the rest of the job, and so the balloon begins to float. The steering at any given direction is probably a different story, but the general concept of the up and down movement can be explained with Charles' law.

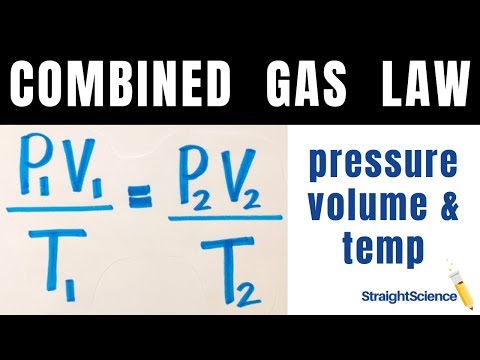
A sample of helium gas in a piston has a volume of 86.4 mL under a pressure of 447 torr. What will be the volume of the helium if the pressure on the piston is increased to 1,240 torr? Assume that the temperature and the amount of the gas remain constant. Which situations does the combined gas law enable you to do calculations when the other gas laws... The line stops at 111 K because methane liquefies at this temperature; when extrapolated, it intersects the graph's origin, representing a temperature of absolute zero. So the only equation you really need to know is the combined gas law in order to calculate changes in a gas' properties.

Since the question never mentions a temperature we can assume it remains a constant and will therefore cancel in the calculation. You should also think about the answer you get in terms of what you know about the gases and how they act. Checking our answer, this appears to be correct since the pressure went from 1atm to 0.6atm. In another lesson, you learned about ideal gases and the ideal gas equation. But since real gases behave similarly to ideal gases at normal temperatures and pressures, we can use the ideal gas equation to predict the behavior of real gases under these conditions. To be more realistic, assume the initial volume of fuel vapour plus air was 400 cm3, now re-calculate the final pressure.

The particle theory of gas pressure was explained in Part 1so this section concentrates on the gas law calculations involving pressure and volume. A sample of nitrogen gas is confined to a balloon that has a volume of 1.88 L and a pressure of 1.334 atm. What will be the volume of the balloon if the pressure is changed to 0.662 atm? Temperature is sometimes measured with a gas thermometer by observing the change in the volume of the gas as the temperature changes at constant pressure. The hydrogen in a particular hydrogen gas thermometer has a volume of 150.0 cm3 when immersed in a mixture of ice and water (0.00 °C).

When immersed in boiling liquid ammonia, the volume of the hydrogen, at the same pressure, is 131.7 cm3. Find the temperature of boiling ammonia on the kelvin and Celsius scales. Plug in the values for initial and final temperature and pressure and the value for initial volume to find the new volume. When you add heat to a liquid, you increase the kinetic and vibrational energy of the particles comprising it. As a result, they increase their range of motion within the limits of the forces holding them together as a liquid. These forces depend on the strength of the bonds holding molecules together and binding molecules to each other, and are different for every liquid.

The coefficient of volumetric expansion -- usually denoted by the lowercase Greek letter beta (β​) --​ is a measure of the amount a particular liquid expands per degree of temperature change. You can look up this quantity for any particular liquid in a table. Although liquids expand/contract on heating/cooling, the volume changes are far less compared to gas volume changes for the same temperature change. This is because of the relatively strong intermolecular forces between liquid molecules, which are almost absent in gases.

Kinetic particle reasoning - increasing the temperature increases the kinetic energy of the molecules giving more forceful collisions which push out the gas at constant pressure. Charles' law describes the relationship between the volume of a gas and its temperature when the pressure and the mass of the gas is constant. It states that the volume is proportional to the absolute temperature. The Charles' law calculator is a simple tool which describes the basic parameters of an ideal gas in an isobaric process. In the text, you can find the answer to the question "What is Charles' law?", learn what the Charles' law formula looks like, and read how to solve thermodynamic problems with some Charles' law examples. If the initial temperature of the air is −10°C and the air warms to 37°C, what is the new volume of the air?

If the initial temperature of the air is 18°C and the air warms to 37°C, what is the new volume of the air? Assume that the pressure and amount of the gas remain constant. The physical properties of gases are predictable using mathematical formulas known as gas laws.

States that the volume of a given amount of gas is directly proportional to its temperature on the kelvin scale when the pressure is held constant. Q. A gas sample has an initial pressure of 571 mmHg and an initial volume of 0.526 L .What is the pressure when the volume of the sample is... Both the ideal gas law and the fact first law for an adiabatic process yield an expression for T3/T1.

We equate these expressions to find the new equilibrium position. Gay Lussac's Law - states that the pressure of a given amount of gas held at constant volume is directly proportional to the Kelvin temperature. Avagadro's Law- Gives the relationship between volume and amount of gas in moles when pressure and temperature are held constant.